Eugene Paul Wigner: Poincar� Group after Einstein

• Of course, there were physicists who worried about relativity applicable to rigid bodies, like rigid spheres or rigid potatoes, and there still may be those worrying about this problem. However, quantum mechanics changed the world. Rigid bodies are now wave packets and standing waves. OK! Do we then understand those wave objects in the Lorentz-covariant world? We can talk about this problem later.

  Before tackling this difficult problem, we must study the basic internal space-time symmetries of relativistic particles. In 1939, Eugene Wigner published an article in Annals of Mathematics while he was at the University of Wisconsin (Madison). This paper had been rejected by three different journals until John von Neumann (then the editor of the Annals of Mathematics) decided to publish it in his journal.

• In this paper, Wigner considers a free particle whose four-momentum is known. Then, are there other degrees of freedom for this particle? The Lorentz group has six degrees of freedom (three boosts and three rotations). If we fix the four-momentum, we are freezing three degrees of freedom (three, not four because the energy is determined by Einstein's energy-momentum relation). What happens to the remaining three degrees of freedom.

  If a particle has its nonzero mass, like the proton or the earth, there is a Lorentz frame in which this particle is at rest. In this case, this particle has three rotational degrees of freedom. These degrees of freedom are associated with the dynamical quantity called "spin."

• In group theory terms, Wigner was constructing the maximal subgroup of the Lorentz group whose transformations leave the four-momentum of the given particle invariant. This subgroup is called Wigner's little group. This little group dictates the internal space-time symmetry of relativistic particles.

• If the particle in question is massless, there are no Lorentz frames in which the particle is at rest. The best we can do is to specify the direction and magnitude of the momentum. It also takes up three degrees of freedom. Thus, the massless particle also has three internal degrees of freedom. In his 1939 paper, Wigner also worked out the little group for massive particle. He showed that rotations around the momentum leaves the momentum invariant. This symmetry is associated with the helicity. Wigner found two additional symmetry operations which leave the four-momentum invariant. From the group theoretical point of view, these symmetry operations are like translations in two-dimensional Euclidean space while the helicity corresponds to the rotation around the origin in this two-dimensional space.

• In his 1939 paper, Wigner was interested in unitary representations of the little groups, and noted that these translation-like operations cannot be associated with measurable quantities. Thus, he suggested restricting representations which freeze these translational degrees of freedom, as we can see in this page of Wigner's paper.

  I started reading Wigner's 1939 paper while I was a student at Princeton (1958-61), but did not completely understand its content until I became much older. Of course, the key issue was what physics can be associated with those translation-like degrees of freedom for massless particles.

  In 1980, D. Han started working with me. We performed a combination of rotations and boosts which would preserve the four-momentum of a massless particle and applied this transformation to the electromagnetic four-vector.

  1. The result was a gauge transformation. This was an exciting moment for us. Indeed, the translation-like degree of freedom in Wigner's little group is a gauge transformation. However, upon studying Weinberg's 1964 papers on the Lorentz group, we became convinced that Weinberg had this idea in 1964 even though he did not explicitly stated so there.

  2. Click here for a more detailed explanation of the E(2) problem.

  3. In 1982, with D. Han and D. Son, I published a paper emphasizing Weinberg's role identifying the translation-like degrees of freedom as gauge transformations.

  4. Again in 1982, we published an article stating that the neutrino polarization is a consequence of gauge invariance.

  5. In 1985, we published a paper stating that, though it is not possible to make unitary boosts transformations on the photon polarization vector, unitary transformations are possible if the boost is accompanied by a little group (gauge) transformation.

  6. In 1986, we published a review paper entitled Photons, Neutrinos, and Gauge Transformations in the American Journal of Physics, for non-experts in the field. I fondly say that this paper is written for children.

• While we were publishing our papers, we noticed the papers which explicitly stated that the four-by-four matrices which performs the translation-like transformations are perform gauge transformations, before my paper of 1981 with Han, but after Weinberg's 1964 papers. They were written by A. Janner and T. Janssen in Physica, Vol.53, page 53 (1971) and Vol.60, page 292 (1972), and by J. Kuperzsytych in Nuovo Cimento B, Vol. 31, page 1 (1976).

  While this was going on, there were some people very unhappy with what we were doing. Their contention was that Weinberg never said the little group transformations are gauge transformations in his 1964 papers. They said further that the translation-like transformation cannot exist in Weinberg's construction, because he starts with the representation with zero eigenvalue of those translation-like operators.

• This opposition was strong enough to affect my professional advancement. This was not the first experience of this kind in my research life. I went through the storm known today as the Dashen-Frautschi fiasco, and I even know how to enjoy them. As is widely known these days, in his 1995 book on on quantum field theory, Weinberg explicitly says translation-like transformations correspond to the gauge degree of freedom.

• The story is not over yet. When we talk about gauge transformations on photons we talk about one degree of freedom. There are two translation-like degrees of freedom in Wigner's little group for photons. How can we collapse those two into one. During the period 1985-91, I used to go to Princeton regularly to tell Professor Wigner the stories he wanted to hear. In 1985, he was 83 years old, but he was eager to write new papers. He was very happy to hear that those two translation-like degrees correspond to gauge transformation. He then raised the question of how two translations become one gauge transformation. We worked hard, and published a paper in 1987 providing a solution to this problem. The point is that the little-group is only iomorphic to the two-dimensional Euclidean group. The little group takes the form of transformations on a cylindrical surface consisting of rotations (helicity) and up-down translations (gauge transformations).

  Many people say Wigner was my thesis advisor when I was a student at Princeton. This was not the case. My advisor was Sam Treiman, who is also known as Weinberg's advisor. Weinberg was four years ahead of me, and I read his thesis before writing mine. I know how to read Weingberg's papers.

  Y. S. Kim (2005.5.12)

Poincar� Group for Extended Particles

Henri Poincar� was born in 1854, 25 years before Einstein. He had a cousin named Raymond who was the president of France (1913-1920). Bertrand Russell of England once said Henri, not Raymond, was the greatest man France had ever produced.

Russell was not the only one who had a great admiration for Henri Poincar�. You must also have an admiration for him in your own way. I also respect him, and I express my admiration in the following way. Since 1973, all of my published and unpublished papers have something to do the Poincar� group. In collaboration with Marilyn Noz, I published a book entitled "Theory and Applications of the Poincar� group." Here is a review of this book written by Marino del Olmo. In addition, I have shown that the Lorentz group, pioneered by Poincar� is the basic mathematical language for optical sciences.

In spite of my life-long dedication to one of his mathematical instruments, I am not the best person to tell you about Henri Poincar� and his role in Einstein's formulation of special relativity. Raymond Streater is not only an outstanding physicist but also a very critical history writer. I would like to invite you to visit his web page on Henri Poincar�. According to Streater, Poincar� formulated the Lorentz-covariant space-time symmetry before Einstein completed special relativity as a new physical theory.

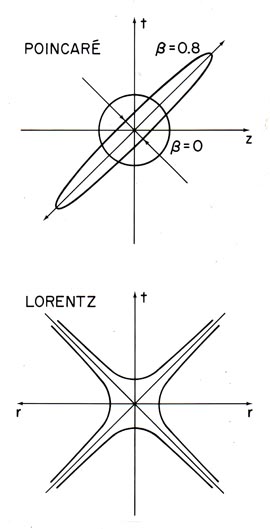
Louis Michel and Eugene Wigner (1984). The difference between the Poincar� and Lorentz groups.

While Poincar� and Lorentz were interested in velocity additions or Lorentz boosts of velocities, Einstein was working on the Lorentz boost of energy and momentum variables. In this way Einstein ended up with his energy-momentum relation.

Then what are the issues after Poincar� and Einstein? The Lorentz group is generated by six generators, namely for three rotations and three boosts. There are two Casimir operators commuting with all of these generators, but there do not seem to correspond to any dynamical quantities.

In the Lorentz-covariant world, we also have to consider space and time translations. There are thus four additional generators, and the total number of generators becomes ten. Poincar� also considered this group and this ten-generator group is called the Poincar� group. Also for this group, there are two Casimir operators which commute with all those ten generators. These Casimir operators correspond to the mass and the spin of the particle.

As Louis Michel pointed out in 1962 in one of his lecture notes, there are confusions in the literature about the difference between the Lorentz group and the Poincar� group. Even these days, the level of confusion remains the same if not worse. In my 1979 paper with Noz and Oh, we were able to amplify Michel's point using harmonic oscillators. I became very happy after learning this difference from Michel.

The Poincar� group was later called "inhomogeneous Lorentz group" by Eugene Wigner in his fundamental paper of 1939. The word "inhomogeneous" was added because the group takes into account space-time translations in addition to Lorentz transformations. In the mathematical language, this inhomogeneous group is a "semi-direct product" of the space-time translation group and the four-dimensional Lorentz group.

Wigner's approach to the Poincar� group is often called "induced representation" by mathematicians, because he starts with subgroups which are called Wigner's little groups. The little group is the maximal subgroup of the Lorentz group whose transformations leave the four-momentum of a given particle invariant.

In this way, Wigner formulated the concept of internal space-time symmetries of relativistic particles. These days, there are many young people interested in inventing a new physics applicable to internal space-time structure of the particle. Their job could become easier if they first study the internal space-time symmetry dictated by Wigner's little groups. I should stop here because I already talked too much about this subject. Click here if you are interested in what I talked about in the past.

Lorentz Group for Optics

This picture is associated with squeezed states of light, but you will see from the ellipse above that it is also a representation of the Poincar� group.

If you studied the polarization of light, you must have seen this sphere, called the Poincar� sphere. Poincar� was quite fond of expressing his ideas using curved surfaces, particularly spherical surfaces.

Poincar� originally constructed this sphere to understand polarization of light waves. These days, it serves as the basic instrument for understanding the density matrix and decoherence mechanism mentioned very frequently in the physics literature.

Did you know that this sphere is a representation of the Poincar�'s Lorentz group? You may be interested in my paper with Sibel Baskal on this subject in Journal of Physics A (2006), or ArXiv.


Since I spent some years on this subject, and I was invited to write a chapter on this subject for a book on mathematical methods in optics. I am now making preparations for writing this Chapter with Sibel Baskal who published many papers with me on this subject. If you think your papers should be mentioned in this Chapter, do not hesitate to contact me at yskim@umd.edu.

The Lorentz group in optics is not restricted to polarizations. The squeezed state in quantum optics is a representation of the Lorentz group. For wave optics, there is the two-by-two matrix called the ABCD matrix. This matrix plays the pivotal role in lens optics, laser cavities, an multilayer optics. Did you know that the ABCD matrix is a representation the Lorentz group? Click here for some of the papers on this subject.

Since both the internal space-time symmetry and optical sciences share the same mathematics, it is possible to study internal space-time symmetries using opticcal instruments. You may be interested in my latest conference talk on this subject.

It is fun to combine optics and particle symmetries to one subject.

Click here for the two-by-two representation of the Lorentz group and its applications.

Who is this young man?

• copyright@2010 by Y. S. Kim, unless otherwise specified.

• Click here for his home page.

• Einstein page.
• Princeton page.

• Style page.
• Travel page.