Lorentz Group in Optical Sciences
Click here for Einstein's photons.
Squeezed States!
Squeezed States!

|
|
Have you seen this picture? It came from my papers. |
The picture is for Lorentz-boosted extended objects in particle physics. The supporting mathematics is called the Lorentz group. This group, by now, is the standard mathematical device for quantum and classical optics.
It is by now widely established that the Wigner function is the key scientific language for quantum optics, but not many people know that those Wigner functions in optics also serve as the representation of the Lorentz groups, such as O(2,1) and O(3,2) which are the fundamental languages for the one- and two-mode squeezed states. When you do squeezed states, you are doing the Lorentz groups.
On this subject, with Marilyn Noz, I have written a book entitled Phase Space Picture of Quantum Mechanics.
Recently, I have been publishing papers on applications of the Lorentz group to classical ray optics, mostly in Phys. Rev. E. Those papers were also archived in Los Alamos. Some of them are listed below.
- Jones matrix formalism as a representation of the Lorentz group
ArXiv. ,
published in J. Opt. Soc. Am. A /Vol.14, No.9 page 2290 (1997).
- Stokes parameters as a Minkowskian four-vector
ArXiv.
Physical Review E (1997). - Illustratative Example of Feynman's Rest of the Universe
Am. J. Phys. [67], 61 - 66 (1999).
Preprint - Wigner rotations and Iwasawa decompositions in polarization optics
ArXiv.
Physical Review E (1999).
- Optics computers for space-time symmetries
ArXiv.
presented at various conferences during the year 1999. - Interferometers and decoherence matrices
ArXiv.
Physical Review E (2000). - Iwasawa effect in multilayer optics
ArXiv.
Physical Review E (2001). - Three-lenses at most in multi-lens system
ArXiv.
Physical Review E (2001) - Wigner rotations in laser cavities
ArXiv.
Physical Review E (2002). -
Lens optics and group contractions
ArXiv.
Physical Review E (2003). - Cyclic representations of multilayer optics
ArXiv.
Physical Review E (2003). - Physics of two-by-two matrices
ArXiv.
presented at various conferences during the year 2003. - Lorentz group in ray optics (Review Paper)
AriXiv.
Journal of Optics B: Quantum and Secmiclassical Optics, Vol. 6, S455-472 (2004). - de Sitter group as a symmetry for optical decoherence
ArXiv.
Journal of Physics A (2006). - ABCD matrices as similarity transformations of Wigner matrices and periodic
systems in optics
J. Opt. Soc. Am. A, 26 3049-2054 (2009). - Possible Minkowskian Language in Two-level Systems
Journal of Optics and Spectroscopy [108], 297-300 (2010).
ArXiv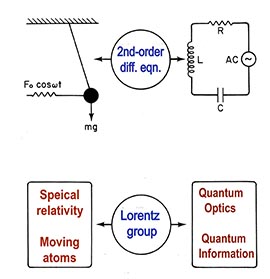
- Optical activities as computing resources for space-time symmetries
Journal of Modern Optics, 57, 17-22 (2010).
-
One anlytic form for four branches of the ABCD matrix,
J. Mod. Opt. [57], 1251-1259 (2010).
ArXiv - Internal space-time symmetries of particles derivable
from periodic systems in optics,
Optics and Spectroscopy [111], 731-737 (2011).
ArXiv. - The Language of Two-by-two Matrices spoken by Optical Devices
19th InternationalConference on Composites or Nano Engineering, Shanghai (China 2011).
AxXiv
with Roy Galuber, Robert Boyd,
Masha Chekova, and Elizabeth
Giacobino (Nuremberg 2103). - Time separation as a hidden variable in the Copenhagen school of quantum mechanics,
in Advances in Quantum Theory,
AIP Conference Series, No.1327, pp 138-147 (2011).
ArXiv. - Lorentz Harmonics, Squeeze Harmonics and Their Physical Applications,
Symmerty [3], 16-36 (2011).
ArXiv. - Poincaré Sphere and Decoherence Problems
Arxiv Based on an invited talk presented at the Fedorov Memorial Symposium: International Conference "Spins and Photonic Beams at Interface," dedicated to the 100th anniversary of F.I.Fedorov (Minsk, Belarus, 2011). - Lorentz Group in Ray and Polarization Optics
Chapter 9 in "Mathematical Optics: Classical, Quantum and Computational Methods" edited by Vasudevan Lakshminarayanan, Maria L. Calvo, and Tatiana Alieva (CRC Taylor and Francis, New York 2013), pp 303-349.
ArXiv. - Symmetries shared by the Poincaré Group and Poincaré Sphere.
Symmetry 5, 223-252 (2013).
ArXiv.
with two big boys from Vienna
(Budapest 2013).
Helmut Rausch (left) and
Anton Zeilinger. - Poincaré Sphere and a Unified Picture of Wigner's Little Groups,
Proceedings of Wigner 111, Colorful & Deep Symposium, edited by
S. Varro, P. Adam, T.S. Biro, G. G. Barnafoldi, and P. Levai,
EPJ Web of Conferences, [78], 01005:1 - 8 (2014),
doi10.151/ejpcon/20147801005.
ArXiv. - Lens optics and the continuity problems of the ABCD matrix
J. Mod. Opt. [61], 161 - 166 (2014).
ArXiv. - Symmetries of Massive and Massless Neutrinos,
Invited paper presented at the International Conference on Hadron Structure and QCD: from Low to High Energies (Gatchina, Russia, 2016).
ArXiv.
- Photons in the Quantum World,
with S. Baskal and M. E. Noz,
Invited paper presented at the 15th International Conference on Squeezed States and Uncertainty Relations (Jeju, South Korea, August 12-17, 2017).
ArXiv. - Mathematical Devices for Optical Sciences.
with S. Baskal and M.E. Noz
IOP Book (2019)
available from the amazon.com - Role of Quantum Optics in Synthesizing Quantum Mechanics and Relativity,
Invited paper presented at the 26th International Conference on Quantum Optics and Quantum Information (Minsk, Belarus, May 2019).
ArXiv. For pdf with sharper images, click here.
| My co-authors. |
| |

|
| Marilyn Noz in 1975 and 2006 |

|
|
|

|
|
Sibel Baskal
|

|
| Elena Georgeiva |
copyright@2021 by Y. S. Kim, unless otherwise specified.
|
- Click here for his home page.
- His photo-biography.
- His Princeton page.
- His Einstein page.
- His Style page.

I received my PhD degree from Princeton in 1961, seven years after high school graduation in 1954. This means that I did much of the ground work for the degree during my high school years.
