E(2)-like Little Group for Massless Particles
For massless particles, Wigner observed the symmetry group is isomorphic to the two-dimensional Euclidean group or E(2), consisting of one rotational and two translational degrees of freedom. It is easy to associate the rotational degree to the helicity. What about the translational degree of freedom? This question was not completely addressed until 1990, 51 years after 1939.- Thus, this is an interesting topic in history of physics. In 1939, Wigner
stated that the symmetry is isomorphic to E(2) and wrote down the matrix of
the form
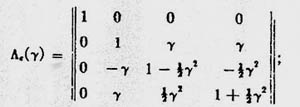
- This is the "ugliest" matrix in physics. Even these days, physicists
give up if the matrix is not unitary. I know this from my
own experience. Since 1973, my papers were based on non-unitary squeeze
matrices. Did you know that Lorentz boosts are squeeze transformations?
As soon as I start telling this, my colleagues run away from me.
It was Steven Weinberg who started tackling the above "ugly" matrix in one of his 1964 papers. In 1979, I studied Weinberg's papers with my younger colleagues, namely D. Han and D. Son, and came up with this figure.
Weinberg talking to Wigner (1957) (top), my interpretation of Weinberg's interpretation of the E(2) symmetry. 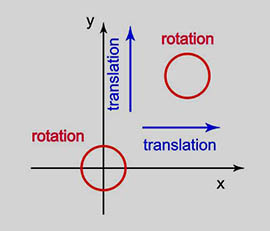
This two-dimensional figure tells about rotations and translations in the E(2) plane, and the translations as gauge transformations. As for the issue of gauge transformations, there are several other authors who said the translations correspond to gauge transformations, and they were quoted in my papers. Most certainly, I am not the first one to observe this aspect of Wigner's E(2)-like symmetry.
- After seeing this, I thought the person who would appreciate this most
was Eugene Wigner who wrote the above "ugly" matrix in his 1939 paper.
In 1985, I went to Princeton to tell him about the translation-like
variables in his E(2)-like group. He became very happy, but asked why
there have to be two gauge components ( x and y coordinates).
I then had to work hard to make Wigner happy. I pulled out Wigner's 1953 paper with Inonu on group contractions, telling that a spherical surface can become flat if the radius becomes large. Thus, we can consider a tangential plane for the E(2) symmetry.
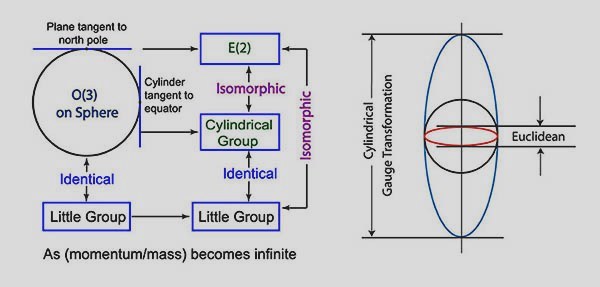
The E(2) symmetry comes from the plane tangential at the north pole, while the cylindrical symmetry comes from the cylinder tangential at the equatorial belt (left figure). Click here for the 1987 paper by Kim and Wigner.
The four-by-four Lorentz transformation matrix produces a geometry which deforms a sphere into an egg and a pancake, which eventually become a cylinder and a plane respectively. Click here for the 1990 paper by Kim and Wigner. - Indeed, both translation-like variables correspond to one up-down translation
on the cylindrical surface. Thus, Wigner's E(2)-like symmetry has one
rotational degree and one translational degree of freedom. This translational
degree of freedom corresponds to the gauge degree of freedom.
During the period of five years (1985-90), I went to Princeton frequently to seek guidance from Eugene Wigner. I was like a graduate student working on his thesis research under Wigner's guidance. When I was a "real graduate student" (1958-61), I was afraid of him.
These days, I am frequently introduced as Wigner's youngest student, while my thesis advisor was Sam Treiman when I got my degree in 1961. I become angry when this introduction is based on my photos with Wigner. I will be very happy if I am so introduced based on the two papers quoted above.
- The above story is based on the following papers.
- E. Wigner, Ann. Math. 40 149 (1939).
- E. Inonu and E. P. Wigner, Proc. Natl. Acad. Sci. (U.S.)
39, 510 (1953).
Click here for further information. - S. Weinberg,
Phys. Rev. 133, B1318 (1964).
- S. Weinberg,
Phys. Rev. 134, B882 (1964).
- S. Weinberg,
Phys. Rev. 135, B1049 (1964).
- A. Janner and T. Jenssen,
-
Physica 53, page 1 (1971), and
Physica 60, page 292 (1972).
- J. Kupersztych,
Nuovo Cimento B 31, page 1 (1976).
- D. Han, Y. S. Kim, and D. Son, Phys. Rev. D 25, 461 (1982).
- D. Han, Y. S. Kim, and D. Son, Phys. Rev. D 31, 328 (1985).
- D. Han, Y. S. Kim, and D. Son,
J. Math. Phys. 27, 2228 (1986).
- Y. S. Kim and E. P. Wigner, J. Math. Phys. 28, 1175 (1987).
- Y. S. Kim and E. P. Wigner, J. Math. Phys. 31, 55 (1990).
Neutrino Polarization
|
Now the question is whether neutrino polarization is a God-given natural law or is derivable from a more fundamental physical principle. Instead of resorting to God, let us ask Einstein. In 1905, Einstein formulated special relativity with the Lorentz group as the fundamental space-time symmetry of particles.
Among the transformations, in his classic paper of 1939, Eugene Wigner considered subgroups of the Lorentz group which leaves the four-momentum of a given particle invariant. They are called Wigner's little groups these days. These subgroups dictate the internal space-time symmetries of particles in the Lorentz-covariant regime.
For a massive particle, Wigner's little group is locally isomorphic to the three-dimensional rotation group. The particle can be brought to the Lorentz frame in which it is at rest. In this frame, rotations leave its four-momentum invariant.
For a massless particle, the little group is isomorphic to the two-dimensional Euclidean group, consisting of rotations around the origin and two translations. It is not difficult to associate the rotational degree with the helicity of the massless particle. What happens to the two translation degrees of freedom. They correspond to gauge degrees of freedom. Wigner's original 1939 paper does not mention this. After a stormy history, it was finally determined in 1990 that these two translational-like degrees of freedom collapse into one gauge degree of freedom. See also the link on Wigner and Poincaré.
For the spin-1 photon, its four-vector potential is gauge-dependent, and is not directly observable. Its electromagnetic field is gauge-invariant and is directly observable.
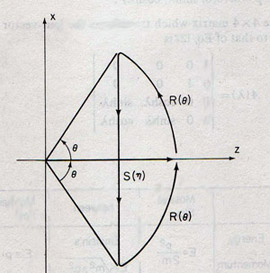
|
| This figure is from Han and Kim, Phys. Rev. A vol. 37, 4494 (1988). |
Of course it is very difficult explain this in the html language without formulas. For a review article, you my visit http://arxiv.org/abs/hep-th/0201262.
- copyright@2014 by Y. S. Kim, unless otherwise specified. Dieter Brill contributed the 1957 photo of Wigner and Weinberg.

| |
|