Heisenberg's Gift to Einstein
E = mc2 derivable from the Poisson brackets
|
is most frequently visited. Since this page is so popular, you are invited to visit this page again. For the same reason, I keep adding new stories about Heisenberg's meeting with Einstein.
- In 1954, Heisenberg went to Princeton to talk with Einstein, and
the meeting lasted longer than scheduled, according to Heisenberg.
However, it was not a successful meeting. After the meeting,
Einstein expressed his displeasure to his personal friend named "Johanna Fantova."
Click here for the story.
- Why did Heisenberg fail to make Einstein happy? The answer is
very simple. He was not able to tell a story Einstein wanted to hear.
Heisenberg was aware that Einstein did not like his interpretation
of the Poisson brackets. However, he could have made Einstein happy
by telling him that the the Poisson brackets lead to the symmetry of
Einstein's special relativity which leads to E = mc2.
He could not tell this story because he was not aware of this in 1954.
It is now possible.

How did this young man become so close to Wigner? He told the stories Wigner wanted to hear. - In 1987, I surprised many people by publishing a paper with Eugene Wigner.
Wigner was known as one of the most difficult persons to approach, and
he was totally isolated from his colleagues at Princeton's physics department.
However, I was able to tell him the story he wanted to hear. The story goes like this.
- Einstein got his Nobel prize in 1921, but not for his mc2.
- It is generally agreed that Einstein deserved one full Nobel for his mc2.
Likewise, Wigner deserved one full prize for his 1939 paper, and I showed him
the following table.
- Wigner got his prize in 1963, but not for
his 1939 paper on internal space-time
symmertries
- I showed Wigner the following table in one of my papers published in 1986.
This table is from one of my papers published in 1986.Contents of Einstein's E = mc2
Particle Massive/Slow between Massless/Fast Einstein Energy
MomentumE = p2/2m E =
[m2c4 + (cp)2]1/2E = cp Wigner Helicity
spin, GaugeS3
S1 S2Winner's
Little GroupsHelicity Gauge Trans. This table made Wigner happy enough to invite me to his office regularly to hear the stories he wanted to hear. Based on those stories, I published a number of papers with Wigner.
- Einstein got his Nobel prize in 1921, but not for his mc2.
- Let us go back to the Heisenberg issue. Heisenberg could have
made Einstein by telling his E = mc2 is a conseqeunce
of the symmetries derivable from his uncertainty commutation relations.
He did not know this in 1954.
This fact was not known until I published a paper in 2019, with Sibel Baskak and Marilyn Noz. You are welcome to read this paper, but I can summarize the result using this webpage.
You may click here for the rotation generator and two squeeze generators for the Sp(2) group corresponding to the limited Lorentz group. You may cliche Click here for detailed calculations.
The symmetry of the Poisson bracket leads to the the Lorentz group appliable to x, z, and t coordinate. How about the y direction? - Thus, in order to get the Lorentz group apllicable to the three-dimensional
space, we can bring in another Heisenberg relation. We noted that the best way to
study was to use the Gaussian form for two-dimensional space of x and p. The Gaussisn
form is of course that for the harmonic oscillator.
Thus, the best way is to increase the dimensision is to use another osicllator. For this two-oscillator system, we need four-dimensional phase space for two pairs of x and p variables. We also need some physics guidelines.
The oscillator form of the Heisenberg relation was most useful in quantum optics. We all know how the coherent state works for the single-photon coherent systems.

More recently, in 1976 (Yuen), two-photon coherent state was introduced, with this generator.
Ten years lalter, in 1986, Yurke, McCall, and Klauder introduced two-photon interferometers. They lead to six more generators.
- We now have seven operators. It is of mathematical interest to see whether they can
form a closed set of commutation relations (called Lie algebra). These operators generate
canonical transformations in the four-dimensional phase space. They can be represented by
four-by-four matrices.
In addition, there are ten five-by-five matrices which can generate Lorentz transformations applicable to three space dimensions (x,y,z) and two time-like dimensions (t,s), or the group O(3,2). This aspect was noticed by Paul A. M. Dirac in 1963.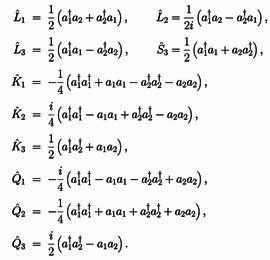
You click on this figure to enlarge it. - In the language of Dirac's O(3,2), there are three rotation generators
for the three-dimensional
space of (x, y, z). In addition, there is one rotation generator for the two time-like
coordinates (t, s). There are thus
four rotation generators. These generators are five-by-five matrices
applicable to (x,y,z,t,s).
There are three boost generators, K1, K2, and K3 with respect to the time-like variable t, and three more: Q1, Q2, and Q3 for boosts with respect to the time-like variable s. These six generators are tabulated in this table.
- Again, according to Dirac,
the fundamental space-time symmetry consist of three rotation generators, three boost
generators, and four translation generators. This symmetry is often called the
Poincaré symmetry or the symmetry of the inhomogeneous Lorentz group IO(3,1). The best
way to accomplish this purpose is to convert Q1, Q2, Q3,
and S3 to the translation generators.
For thais purpose, we use another concept developed in quantum optics, namely
Squeeze.
In the language of group contractions,
These generators lead to the following translations in the (x,y,z,t) coordinates:
In this way, Dirac's O(3,2) become contracted to IO(3,1). Diriac's O(3,2) is derivable from the symmetry of the Poisson brackets.
- In 2019, I published the following papers telling that Einstein's E = mc2
can be derived from the Heisenberg brackets.
- Einstein's E = mc^2 derivable from Heisenberg's Uncertainty Relations,
with Sibel Baskal and Marilyn Noz,
Quantum Reports [1] (2), 236 - 251 (2019),
doi:10.3390/quantum1020021.
ArXiv. For pdf with sharper images, click here. - Role of Quantum Optics in Synthesizing Quantum Mechanics and Relativity,
Invited paper presented at the 26th International Conference on Quantum Optics and Quantum Information (Minsk, Belarus, May 2019).
ArXiv. For pdf with sharper images, click here. - Poincaré Symmetry from Heisenberg's Uncertainty Relations,
with S. Baskal and M. E. Noz,
Symmetry [11](3), 236 - 267 (2019),
doi:10.3390/sym11030409.
ArXiv.
- Einstein's E = mc^2 derivable from Heisenberg's Uncertainty Relations,
- copyright@2018 by Y. S. Kim, unless otherwise specified.
- Click here for his
home page.
- Einstein page.
- Princeton page.
- His stories. He likes
to tell you stories.

| |
|



