Dirac's Efforts to synthesize Quantum Mechanics and Special Relativity
|
- It took many agonizing years for physicists to formulate quantum mechanics,
but we say quantum mechanics was born in 1927 when Heisenberg gave his
interpretation to the Poisson brackets. They act as the bridges between
classical mechanics and quantum mechanics, and also between the particle
nature and wave nature of matter.
The question is whether the uncertainty brackets serve as the bridge between quantum mechanics and special relativity.
- In 1927, Driac started worrying about whether this new theory is
consistent with special relativity. He then made continued efforts
to make quantum mechanics consistent with Einstein's special relativity
or Lorentz covariant. Among the many papers he wrote on this subject,
he made his original contributions in the following four papers.

Unlike Heisenberg, Dirac was interested in whether Heisenberg's brackets are consistent with special relativity. - Dirac (1927).
The Quantum Theory of the Emission and Absorption of Radiation,
Proc. Roy. Soc. (London) A [114], 243 - 265 (1927).
- Dirac (1945).
The Quantum Theory of the Emission and Absorption of Radiation,
Proc. Roy. Soc. (London) A [A183], 284 - 295 (1945).
- Dirac (1949).
Forms of Relativistic Dynamics,
Rev. Mod. Phys. [21] 392 - 399 (1949).
- Dirac (1963). A Remarkable Representation of the 3 + 2 deSitter Group, J. Math. Phys. [4], 901 - 909 (1963).
Let us consider these four papers. They are original and deep-penetrating. No ordinary physicists could write papers like these. This does not necessarily mean that there is nothing to add to them.
- Dirac's papers are like poems. It is a pleasure to read them. However,
there are no figures, unlike Feynman who was a cartoonist (Feynman diagrams are
cartoons). Let us compare
Dirac and Feynman. Click here.
It is thus fun to translate Dirac's poems into cartoons.
- In his papers, Dirac never mentioned his earlier papers on the same subject.
In
his paper of 1945, Dirac introduced the time variable in his harmonic
oscillator wave functions, but he did not mention the difficulty of
incorporating the c-number time-energy uncertainty in the Lorentz-covariant
world which he pointed out in his earlier
paper of 1925.
By talking about oscillator wave function, Dirac was talking about localized waves for bound states. For this system, Wignr's little group is O(3), namely the three-dimensional rotation group. This takes care of the c-number nature of the time-energy uncertainty relation. Click here for an explanation.

Integration of Dirac's 1927, 1845, and 1949 papers.
Ten generators of the O(3,2) group. Is it possible to convert these to the ten generators of the inhomogeneous Lorentz group?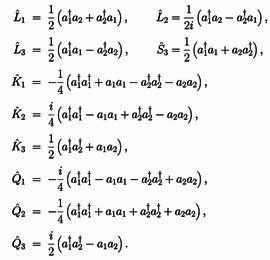
- In
his 1949 paper, Dirac introduced his light-cone coordinate system. This paper
tells that the Lorentz boost is a squeeze transformation.
It is thus fun to combine this squeeze transformation with the oscillator wave function he introduced in his earlier paper of 1945.
Under this squeeze transformation, the circle gets deformed into an ellipse. It is even more fun to see whether this elliptic deformation is observable in the real world. Click here for a story.
- In his 1963 paper,
Dirac constructed the O(3,2) deSitter group from two coupled oscillators. This group is
Lorentz group applicable to three space coordinates and two time variables. Compared with
the Lorentz group of O(3,1), this group has an extra time variable.
Earlier in 1949, Dirac said that the task of constructing the quantum mechanics in the Lorentz-covariant world is to construct a representation of the inhomogeneous Lorentz group, consisting of three rotations, three boosts, and four translations.
- Dirac (1927).
The Quantum Theory of the Emission and Absorption of Radiation,
Proc. Roy. Soc. (London) A [114], 243 - 265 (1927).
- Thus, it is fun to see whether it is possible to obtain the inhomogeneous Lorentz group
mentioned in his 1949 paper from the O(3,2) group he obtained from two coupled
oscillators. Indeed, this is possible. The key is to flatten one of the time
variables to make four translation variables, separating O(3,2) into O(3,1) and
four translations. This process is called the
group contraction introduced by Inonu and Wigner in 1953.
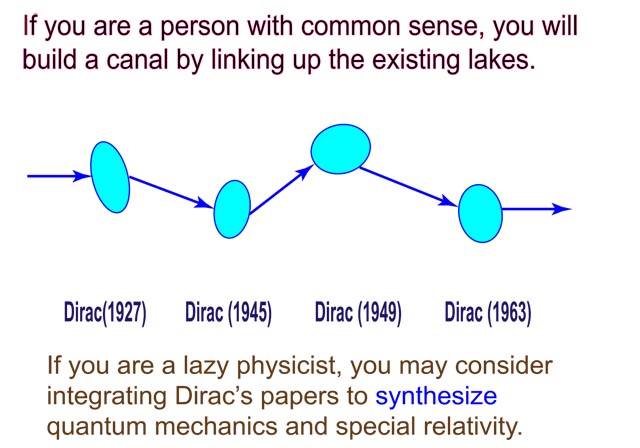

- We thus arrrive at a serious conclusion. The special relativity, with Einstein's
E = mc2, contained
in the inhomogeneous Lorentz group is derivable from the Heisenberg brackets.
Have you heard this story before?
Click here for a published article on
this subject.
We discuss coupled harmonic oscillators in undergraduate physics classes. We never expected this toy could play a role in combining quantum mechanics and special relativity into one scientific discipline. According to philosophers, this is a Hegelian synthesis.
- In 2019, I published the following papers telling that Einstein's E = mc2
can be derived from the Heisenberg brackets.
- Einstein's E = mc^2 derivable from Heisenberg's Uncertainty Relations,
with Sibel Baskal and Marilyn Noz,
Quantum Reports [1] (2), 236 - 251 (2019),
doi:10.3390/quantum1020021.
ArXiv. For pdf with sharper images, click here. - Role of Quantum Optics in Synthesizing Quantum Mechanics and Relativity,
Invited paper presented at the 26th International Conference on Quantum Optics and Quantum Information (Minsk, Belarus, May 2019).
ArXiv. For pdf with sharper images, click here. - Poincaré Symmetry from Heisenberg's Uncertainty Relations,
with S. Baskal and M. E. Noz,
Symmetry [11](3), 236 - 267 (2019),
doi:10.3390/sym11030409.
ArXiv.
- Einstein's E = mc^2 derivable from Heisenberg's Uncertainty Relations,
- copyright@2019 by Y. S. Kim, unless otherwise specified.
- Click here for Y. S. Kim's home page.

|
| Why is he with Einstein? |
