Quasisymmetry

In a stellarator, even if the magnetic field lines lie on nested toroidal surfaces (“flux surfaces”), some particles may still not be confined. Charged particles do drift off of field lines even in the absence of collisions and turbulence, associated with the fact that the magnetic field B must have gradients. Stellarator shapes must therefore be optimized to ensure these cross-field drifts do not allow particles to travel out of the confinement region. A solution to this optimization problem is provided by quasisymmetry, a remarkable property of some magnetic fields that ensures the drift across flux surfaces has a time average of zero.

To understand qasisymmetry, first consider axisymmetry, meaning standard continuous rotation symmetry. In a truly axisymmetric magnetic field, conservation of canonical angular momentum ensures that the drift across surfaces averages to zero over time, so a particle’s departure from a particular magnetic surface is small and bounded. But this cross-field drift can also average to zero in the absence of true axisymmetry, due to a remarkable result: once the fast gyration of particles about B is averaged over, canonical angular momentum conservation requires only a continuous symmetry of B=|B|, the magnitude of the field, not of the full vector B. Such a symmetry of B is called ‘quasisymmetry’. Non-axisymmetric magnetic fields, even if they are integrable, are generally not close to being quasisymmetric. However, using optimization, magnetic fields have been found that lack true axisymmetry but approximately possess both integrability and quasi-symmetry. One stellarator of modest size has been built on this principle, the Helically Symmetric Experiment (HSX) at the University of Wisconsin, and it has experimentally demonstrated the benefits of quasisymmetry.

Figure 1 shows an example of a quasisymmetric magnetic field. Figure 2 shows the same configuration along with the electromagnetic coils that produce the field.

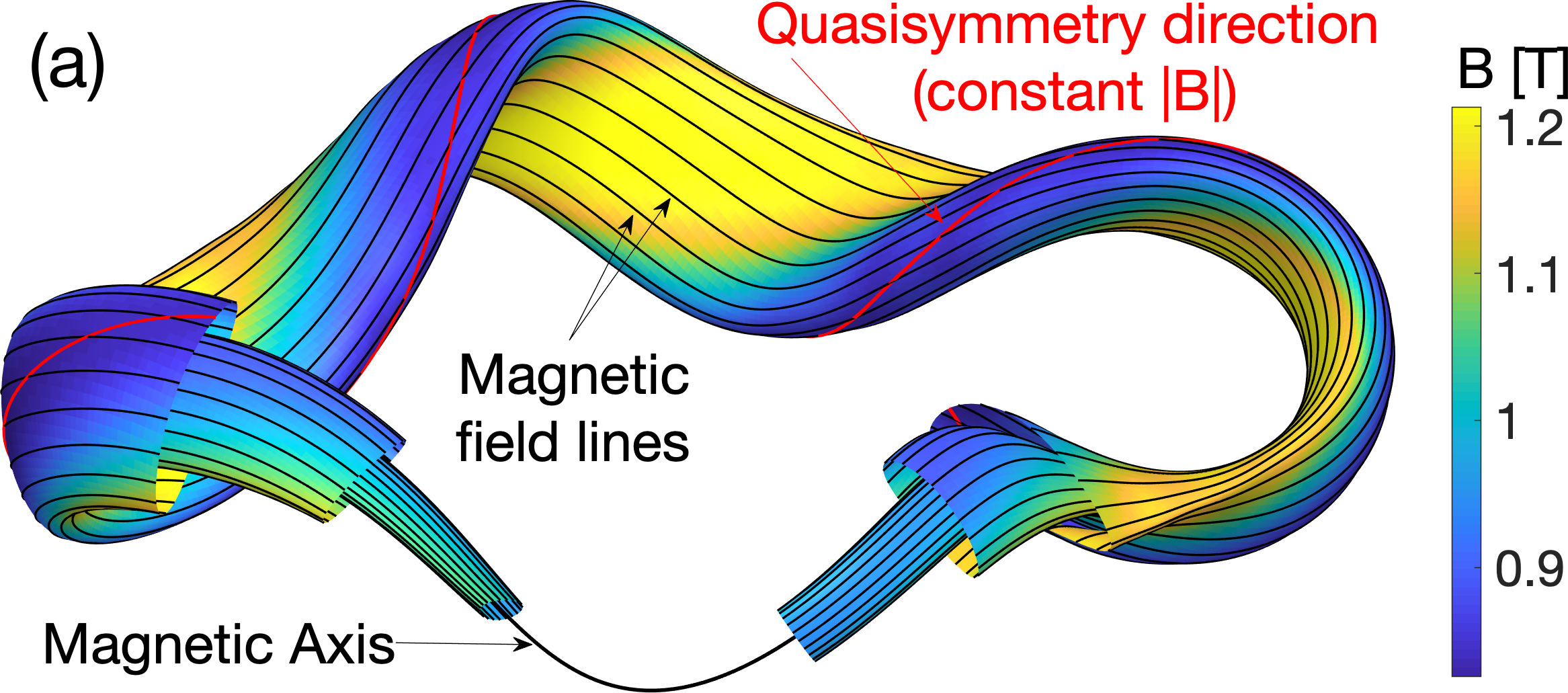
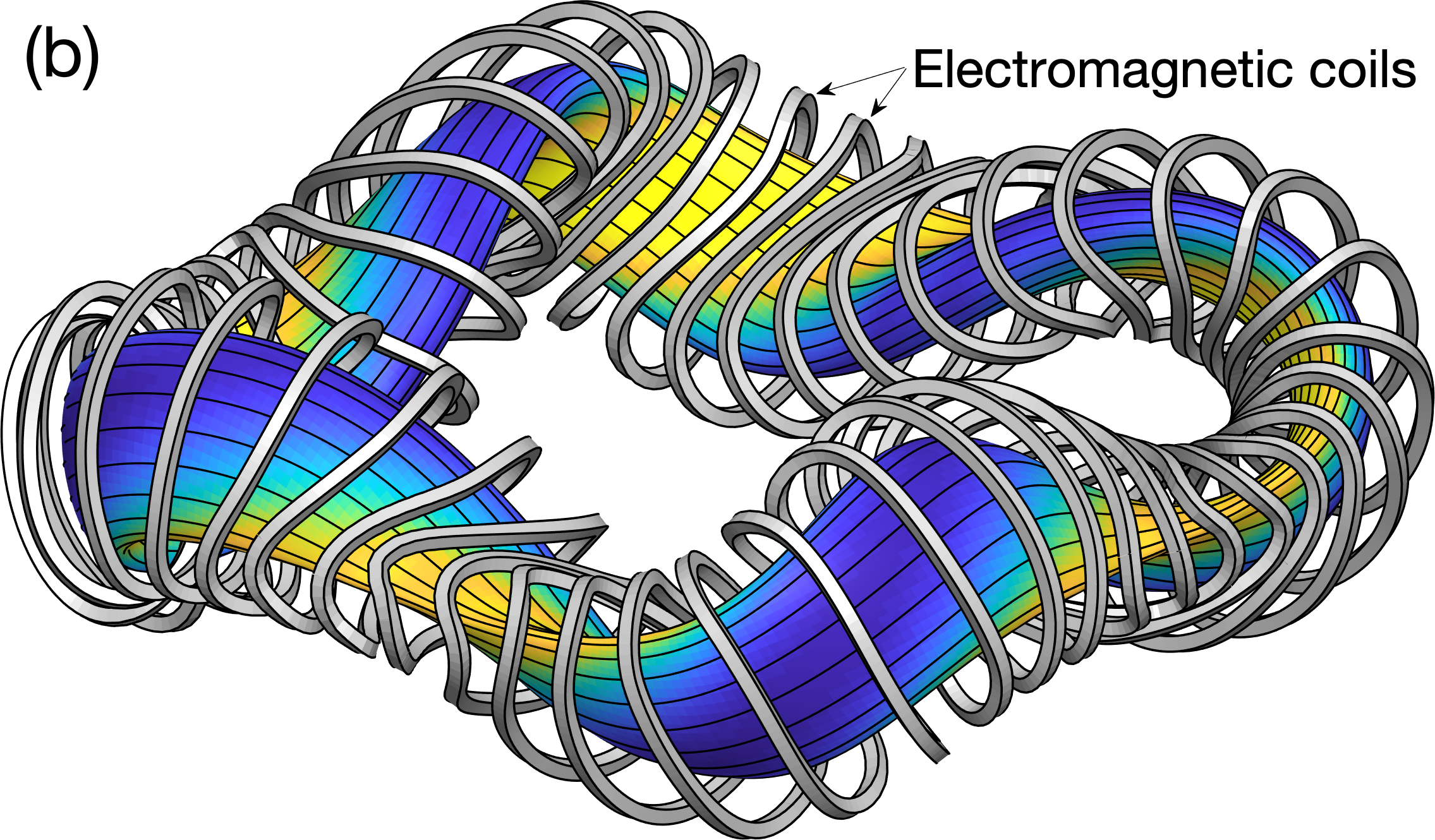
A more detailed introduction to quasisymmetry can be found here.

Our group has developed a new method to find quasisymmetric fields, a method that is about 10⁷x faster than the conventional approach based on optimization. In the new method, we focus on the region close to the magnetic axis, which is the innermost magnetic field line, shown in figure 1. The equations of magnetohydrodynamic equilibrium and quasisymmetry can then be expanded in a small parameter: the distance from the magnetic axis compared to the scale length of the axis (e.g. a typical radius of curvature). This expansion, which is sometimes called a high-aspect-ratio approximation, represents the fact that the core of a stellarator looks more like a skinny bicycle tire than a fat monster truck tire. This expansion is accurate in the core of any stellarator, and it allows the equations of quasisymmetry to be solved much faster. Our new method to find quasisymmetric fields is described here and here, and other details of the method are discussed in papers here, here, and here. Our code implementing this method is available here. This work was done with our collaborators at NYU and the Max Planck Institute for Plasma Physics.

Our group is presently researching several topics related to quasisymmetry. Exploiting the speed of our new method for finding quasisymmetric fields, we are numerically surveying the landscape of possible solutions. We are studying analytic properties of the quasisymmetry equations that result from the large-aspect-ratio expansion described above. Using both analytic and numerical methods, we are working to understand how accurately quasisymmetry needs to be acheived. We are also formulating other new methods to find quasisymmetric fields by optimization, different from past approaches in that our methods provide analytic derivatives. Overall, our long-term goal is to identify and describe all experimentally realizable classes of quasisymmetric fields.