Story Einstein wanted to hear from Heisenberg
Among the webpages I built on the history of physics, the page entitledis most frequently visited. This is a follow-up on this popular page.
- In 1954, Heisenberg went to Princeton to talk with Einstein, and
the meeting lasted longer than scheduled, according to Heisenberg.
However, it was
not a successful meeting.
The meeting was not successful because Heisenberg could not tell
what Einstein wanted to hear.
- In 1954, it was not possible for Heisenberg to tell Einstein that the
symmetry of his Lorentz covariance, resulting in E = m2,
can be derived from Heisenberg's uncertainty commutators.
- It is now possible to tell Einstein that the symmetry of the Lorentz covariance
is derivable from the symmetry of Heisenberg uncetainty relations. Here is a paper
published paper on this subject.

- The momentum operators Heisenberg's commutation relations generate translations.
Together with the coordinate variables, they lead to three rotation generators.
This allows us to construct three rotation genenerators.
These three translation and three rotation genetaros constitute the symmetry goup
of the quantum mechanics in the Galiaan world, as Wigner noted in 1933.
- In 1949, Paul A. M. Dirac noted that the time variable can be augmented the Galian
system, and he addeded the three Lorentz-boost generators and one translation along
the time variable. There are thus three rotation generators, three boost generators,
and four translation generators.
- In 1963, Dirac noted that Heisenberg's uncertainty relation can written in terms of
the step-up and step-down operators for the oscillator system. For a single osicllaor
system, the commuatrion relations can be written as
- Let us go back to the one-variable commutation relation. This commutation relation
contains shares the same symmetry property as that of the classical Poisson bracket.
This problem can be addressed most effectively with the Wiger distriution function
which takes the form
Here we can rotate in the two dimensional phase space without changing the normalization of the Gaussian function. We can also squeeze the coordinate, by making x larger while making p smaller. The net result is the transformation group consisting of the rotation, squeeze along the x and P coordinate, and squeeze along the 45o direction. There are three generators satisfying the closed set of commutation relations which is the same as the set for the Lorentz group applicable to Lorentz boost along the x, and z directions and the rotation around the y direction.
You may click here for the generators in the two-dimensional phase space and corresponding four-by-four matrices applicable to the four-dimensional Mikowskain space. Click here for detailed calculations.
- The problem is how to expand this group to include an additional space-like
coordinate. Use one more Heisenberg brackets. How? For this
purpose, we can write Heisenberg commutation relation in terms of the step-up and
step-down operators.
- You may also be interested in the webpage entitled
Dirac's poems and Feynman's drums.
-
It is then possible to construct three rotation generators
satisfying the commutation relations
Let us go to the Old Testament of the Bible. In order to get the family right, Jacob tells a wrong story to his father to make him happy. Why didn't Heisenberg bring a story Einstein wanted to hear? Many people are wondering how I became so close to Eugene Wigner during his late years. The answer is very simple. I was able to tell Wigner the stories he wanted to hear. My grandfather was a landlord. I was in competition with my cousin for inheriting his fortune, and I had to invent stories my granddaddy wanted to hear. This childhood training worked for Wigner.
- Here comes the key question. Did you know the Poisson brackets
lead to the Lorentz group? Don't worry. I am not the first person
who observed this. It was Paul A. M. Dirac who used two harmonic
oscillators to derive the group of Lorentz transformations. Dirac
in fact derived two coupled Lorentz groups or O(3,2) group starting
from two oscillators.
Dirac published this result in 1963 in the Journal of Mathematical Physics. This paper is largely unknown to the present generation of physicists, in spite of the fact that it provides the mathematical base for many branches of physics, including squeezed states, entanglements, entropy, high-energy physics, Bogoliubov transformation in superconductivity, O(3,2) supersymmetry, and presumably many future theories..
Both Dirac and Heisenberg were interested in the Poisson brackets. Einstein did not like Heisenberg's interpretation of those brackets. On the other hand, Dirac was interested in extending those brackets to Einstein's Lorentz-covariant world.
Dirac's 1963 paper contains the ten generators of the Sp(4) group isomorphic to the O(3,2) Lorentz group. He constructed them from two harmonic oscillators or two Poisson brackets. Click here for those generators.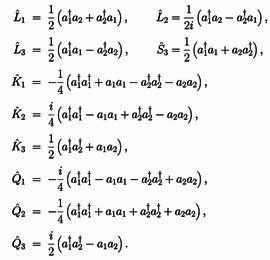
You may click here to enlarge this figure. You may also be interested in a paper where the O(3,2) symmetry is expanded to the O(3,3) symmetry with 15 Dirac matrices. Click here for the paper published in J. Math. Phys. [36], 3940-3954 (1995). The symmetry of the Poisson bracket leads to the the Lorentz group - Then, while you did not know, how do I know about this paper? In
the fall of 1962, spent many hours with Dirac. How did this happen?
Click here for a story. At
that time, I did not like what was going on the physics world.
Click here to see how much I disliked
the physics environment at that time. I had to be born again, like
Nicodemus after seeing Jesus (Bible story from the Gospel of John).
Dirac's 1963 paper is difficult to read, because it consists of a mathematical poem consisting of ten generators and thus sixty commutation relations. It is fun to provide interpretations to this poem using physical examples, and this has been my research line for many years.
- Then, what does this have to do with Heisenberg? If Dirac got the
Lorentz group from the commutation relations for harmonic oscillators,
there must be the basic element of Lorentzian symmetry in Heisenberg's
Poisson brackets. Indeed, this symmetry is well known, and it is called
the group of canonical transformations.
The Poisson bracket consists of two conjugate variable x and p.
This bracket is invariant under rotations in the phase space of those two
variables. It is also invariant when x increases while p decreases while
the product xp remains constant. This is a squeeze in phase space. These
operations are enough to construct the Lorentz group applicable to the two
space-like and one time-like directions.
This symmetry is not rich enough address the symmetries in the Minkowskian space of three space-like and one time-like dimensions. Dirac in 1963 was able to construct his richer symmetries using the symmetry of two Poisson brackets. Yes, Einstein could have shown his interest in this symmetry of the Poisson brackets.
- The rotation in the phase space of x and p leads to the rotation around
y axis in the three-dimensional space. The squeeze along the x~p
directions leads to the Lorentz boost along the z direction. The squeeze
along the 45-degree direction corresponds to the Lorentz boost along the
x direction as shown in this figure.
The following table shows how the generators of the canonical transformations lead to those of the Lorentz transformations in the Minkowskian space of (x, y, z, t).
- This page reflects my strong Christian background. You will not
be surprised to hear about my oriental background. Why is it possible
for me to have a Christian background?
Click here for a story. There are of course pages reflecting
my oriental background. Here is
one of them.

|
- In 2019, I published the following papers telling that Einstein's E = mc2
can be derived from the Heisenberg brackets.
- Einstein's E = mc^2 derivable from Heisenberg's Uncertainty Relations,
with Sibel Baskal and Marilyn Noz,
Quantum Reports [1] (2), 236 - 251 (2019),
doi:10.3390/quantum1020021.
ArXiv. For pdf with sharper images, click here. - Role of Quantum Optics in Synthesizing Quantum Mechanics and Relativity,
Invited paper presented at the 26th International Conference on Quantum Optics and Quantum Information (Minsk, Belarus, May 2019).
ArXiv. For pdf with sharper images, click here. - Poincaré Symmetry from Heisenberg's Uncertainty Relations,
with S. Baskal and M. E. Noz,
Symmetry [11](3), 236 - 267 (2019),
doi:10.3390/sym11030409.
ArXiv.
- Einstein's E = mc^2 derivable from Heisenberg's Uncertainty Relations,
- copyright@2018 by Y. S. Kim, unless otherwise specified.
- Click here for his
home page.
- Einstein page.
- Princeton page.
- His stories. He likes
to tell you stories.

| |
|
