Photons: Maxwell, Minkowski, Einstein, and Wigner

|
|
- Among the Greeks scientists, Aristotle had an idea that the
light has a finite velocity, and Galileo later showed that the
velocity s finite.
Christiaan Huygens(1629-1695) and Isaac Newton (1642-1726) were contemporaries with 53 overlapping years. Huygens said the light consists of waves, while Newton insisted the light beam consists of particles.
In their book, Jenkins and White talk about Young's double-slit experiment and Fraunhoffer diffraction.
-
Maxwell and Minkowski.
photos from the public domain.Marconi. from the public domain.

- Let us become more serious. Maxwell theoretically discovered
electromagnetic radiation. The light waves are now electromagnetic waves.
- Marconi showed that Maxwell's waves can be used for radio
communication. Click here
the first stage of the wireless digital age.
- In 1908, Minkowski proved Maxwell's equations are covariant
under Lorentz transformations, thus are consistent with
Einstein's special relativity.
Click here for more about Hermann Minkowski.
At the Eidgenössische Polytechnikum (ETH Zurich today), he had a
student named Albert Einstein.
- Even though Minkowski published his paper in 1908, it is believed that
Einstein was aware of Minkowski's result that Maxwell's theory is
Lorentz-covariant, while Newton's equation is
not. He saw the
lack of harmony in transformation laws.
Einstein solved this problem by introducing his special relativity in 1905. While Lorentz and Poincaré were interested in the Lorentz transformation of space and time variables, Einstein thought of the same transformation law of the momentum-energy variables. He then ended up with the hyperbolic relation

-
Lack of Harmony! Einstein noted.

-
Einstein in 1905. Photo from the public domain.
-
m2 c4 =
E2 - p 2c2 ,
which is widely known as Einstein's E = mc2 .
Also in 1905, Einstein discovered the photo-electric effect showing that the light can be regarded as a collection of massless particles called photons, and that the photon energy is proportional to the frequency of the light. Click here for a very comprehensive exposition of this important historical event.
Since the photon can be regarded as both wave and particle, it is particle defined in quantum mechanic.
In the wave picture, the Maxwell theory tells the electric and magnetic fields are perpendicular to each other, and they both are perpendicular to the direction of propagation. This picture is Lorentz-invariant.
In quantum world, the photon spin is one and is either parallel or anti-parallel to is momentum. Unlike a slow massive particle, the photon does not have angular momentum perpendicular to it momentum.

Lincoln Wonfenstein was one of my teachers while I was an undergraduate student at Carnegie Mellon University (1954-58). This photo was taken at the MIT faculty club in 1997. - Marconi showed that Maxwell's waves can be used for radio
communication. Click here
the first stage of the wireless digital age.
- When I was a student, I asked my teachers the following questions.
- Why doesn't the photon have the angular momentum perpendicular to
its momentum? The teachers' answer was that it is because the photon
is massless.
- While I was a graduate student, I learned neutrinos are polarized. The neutrino can have its 1/2 spin either parallel or anti-parallel to its momentum, Thus, my question was why the photon can have both helicities while neutrino can have only one. The usual answer given to me was that neutrinos are spin-1/2 particles while the photon spin is one.
Of course, my teachers were all respected physicists. This does not necessarily mean that I was happy with their answers. The best way is to find them out by myself.
- Why doesn't the photon have the angular momentum perpendicular to
its momentum? The teachers' answer was that it is because the photon
is massless.
- In order to provide the answers to these questions, I had to go through
the following steps.
- When I was a graduate student, I had to work on dispersion relations
for the two-particle collision ending up with three particles. I did
not know how to approach the kinematics of this process in the
Lorentz-covariant world. I asked Wigner what to do, even though he was
not my thesis advisor.
He said there are six degrees of freedom in the Lorentz-covariant world. If the total momentum is fixed freezing three of the six degrees of freedom, there are three remaining degrees of freedom.
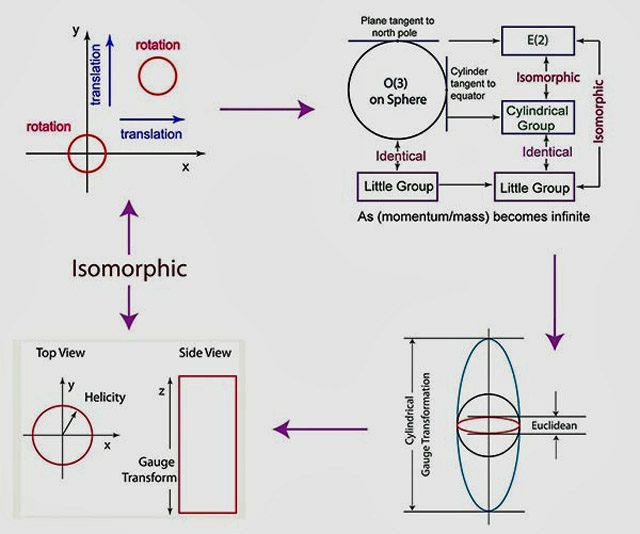
At that time, I did not realize he was talking about his 1939 paper. In this paper, Wigner considered the subgroups of the Lorentz group whose transformations leave the momentum of a given particle invariant. Wigner called them the "little groups." Each little group thus has three degree of freedom. I was not aware of Wigner's 1939 paper at that time.
- Also while I was a graduate student, I and my classmates were all
excited about Feynman diagrams and quantum electrodynamics.
I overheard, at a tea time, Wigner saying to someone "nobody understands
massless particles."
Again, I did not realize he was talking about his little groups. He was
talking about the little group for massless particles.

Physics faculty photo of 1963 at the Univ. of Maryland. I am at the center of the second row. - In 1962, I became an assistant professor at the Univ. of Maryland, I
had to struggle to find my own coordinate in physics. On this issue, I
have a long story to tell, but should not talk too much about myself.
You may click here to see why I had to study
Wigner's little groups which dictate the internal space-time symmetries of
particles in the Lorentz-covariant world.

Wigner with his daughter at the
departmental picnic in Princeton
(1961).
I had a photo with Wigner's daughter
in Princeton (2002).
- When I was a graduate student, I had to work on dispersion relations
for the two-particle collision ending up with three particles. I did
not know how to approach the kinematics of this process in the
Lorentz-covariant world. I asked Wigner what to do, even though he was
not my thesis advisor.
- I then realized Wigner was talking about
his 1939 paper on the following issues when I was a graduate
student in 1959.
- For a given relativistic particle, there are three internal degrees
of freedom when its momentum is fixed.
Rotations do not change the particle momentum if it is at rest.
Indeed, there are three spin degrees of freedom for a particle at rest.
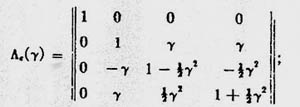
This ugly matrix is on page 165 of Wigner's 1939 paper. This matrix performs a gauge transformation when applied to the electromagnetic four-vector.
Did you know this matrix is a Lorentz-boosted rotation matrix in the infinite-momentum limit? Like to know?
-
For a massless particle, there are also three internal degrees of freedom, with the symmetry
of the two-dimensional Euclidean group, with one rotational and and two
translational degrees of freedom. The rotational degree of freedom is
easy to understand as the helicity.
However, for the translational-like degrees of freedom, Wigner wrote down this strange matrix on page 165 of his paper. Since this matrix is so strange, it used to scare away young physicists from Wigner's paper, and many people used call it "Wigner's ugly matrix."
When Wigner said nobody really understands massless particles when I was a student, he was talking about his ugly matrix for which he was not able to give physical explanations in his 1939 paper.
- For a given relativistic particle, there are three internal degrees
of freedom when its momentum is fixed.
Rotations do not change the particle momentum if it is at rest.
Indeed, there are three spin degrees of freedom for a particle at rest.
- Things are somewhat different these days. It is by now known to many informed
physicists that this matrix performs
a gauge transformation when applied to the electromagnetic four-vector, but not
many of you know that this is a Lorentz-boosted rotation matrix.
The question then is who was the first person to find out this is a gauge-transformation matrix? Don't worry. I am not the person. Then who was the first person?

In this photo of 1957, Weinberg is talking to Wigner. When I went to Princeton in 1958, he was not there. He got his degree in 1957 and went to Columbia University in New York. Another prominent person in this photo is Daniel Sperber. He was still there in 1958. As a senior graduate student, Sperber told me what to do and what not to do as a graduate student. In 2003, we met a conference in Princeton honoring John A. Wheeler. Together, we had this photo. Weinberg from Texas sent a message of appreciation to Wheeler.
The 1957 photo of Wigner, Weinberg, and Sperber was taken by Dieter Brill who also was a graduate student then. Here is a photo of myself with him taken in 1986. Wigner was in the middle.
- When I published my paper on this subject with D. Han in 1981,
I sent the manuscript to the
American Journal of Physics (for physics teachers) instead of
the Physical Review, because we did not think we were the first ones to
see this.
First of all, we were inspired by the following papers published by Steven Weinberg in 1964.
- S. Weinberg,
Phys. Rev. 133, B1318 (1964).
- S. Weinberg,
Phys. Rev. 134, B882 (1964).
- S. Weinberg, Phys. Rev. 135, B1049 (1964).
The abstract of our first paper says
Based on Weinberg’s work on Lorentz-transformation properties of massless particles, we discuss the little group for photons and gauge transformations from a pedagogical standpoint. It is pointed out that the "translational" degrees of freedom associated with the photon little group can generate a transformation which guarantees the transversally of the four-vector representation for photons. This little-group transformation, which leaves the photon momentum invariant, can be regarded as a gauge transformation.
- S. Weinberg,
Phys. Rev. 133, B1318 (1964).
- In 1982, with D. Han and D. Son, I published another paper in PRD with the
following abstract.
It is shown that the photon spin is associated with the rotational invariance in a two-dimensional space spanned by the two "translation" parameters of the E(2)-like little group of the Poincaré group. Since the translation in the E(2)-like space is a gauge transformation, the photon spin can be associated with an orbit-like rotation with nonzero radius in the gauge space. Click here for the paper.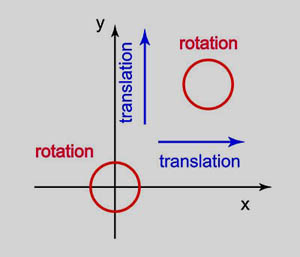
Wigner's symmetry of massless particles isomorphic to the two-dimensional Euclidean group.
- When I published my paper on this subject with D. Han in 1981,
I sent the manuscript to the
American Journal of Physics (for physics teachers) instead of
the Physical Review, because we did not think we were the first ones to
see this.
- After publishing a number of papers on this issue, we found out
A. Janner and K. Jenssen pointed out the ugly matrix performs gauge
transformations, while studying electromagnetic waves propagating in
solid, and published their papers in
-
Physica 53, page 1 (1971), and
Physica 60, page 292 (1972).
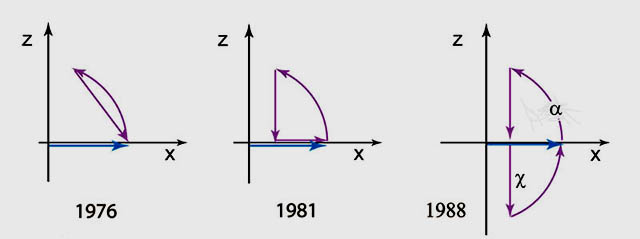
-
In 1976, Kupersztych considered a momentum-preserving transformation
consisting of a rotation followed by a boost. When applied to the
electromagnetic four-vector, this loop transformation performs a gauge
transformation.
Click here
for the evolution of this concept of loop and its role for studying
Wigner's little groups for internal space-time symmetries of massive
and massless particles.
In 1976, J. Kupersztych published a paper, in Nuovo Cimento B 31, No. 1, saying the ugly matrix performs a gauge transformation. The content of his paper is essentially the same as that of our 1981 AJP paper mentioned above. He did before we did.
We were not able to recognize these earlier papers because they did not make references to Wigner's 1939 paper. We of course mentioned their contributions in our later publications. I am very happy to mention their papers here again.
- I continued working on this subject with my younger colleagues. In 1983,
we found out that Wigner's ugly matrix is a Lorentz-boosted rotation
matrix, and the rotations perpendicular to the momentum become gauge
transformations.
Click here for the paper. This paper thus shows
- why the massless particle cannot have its spin perpendicular to
the momentum, and
- the rotation perpendicular to the momentum becomes a gauge transformation.
We continued our work along this line, and published in 1986 a paper in the Journal of Mathematical Physics This paper contained the following table.
Massive/Slow between Massless/Fast Energy
MomentumE=p2/2m Einstein's
E=(m2 + p2)1/2E=p Helicity
Spin, GaugeS3
S1 S2Wigner's
Little GroupS3
Gauge Trans.We constructed this table before 1985. In 1985, I went to Princeton to show this table to Wigner. He became very happy and told me he was eager to write new papers on this subject. When I explained the result in terms of the two-dimensional geometry given in our paper of 1982, he wanted to have the gauge transformation along one direction, not two as illustrated in this picture shown above. We thus worked hard and came up with the following result.
The Euclidean plane can be tangent to a sphere at its north pole. Then there is a cylinder tangent to this sphere at its equatorial belt. The symmetry on the surface of this cylinder is isomorphic to that of the tangent plane. The radius of this sphere becomes very large if the particle speed reaches the light speed.
We then published these results in 1987 and in 1990 in the Journal of Mathematical Physics. Lawrence Biedenharn was the chief editor of this journal. He said he was very happy to publish these papers and congratulated me on re-activating Wigner's 1939 paper. Click here for a more detailed story.
-
Lawrence Biedenharn with Wigner in 1988, during the Wigner Symposium
held at the University of Maryland.
- why the massless particle cannot have its spin perpendicular to
the momentum, and
- Let us write some formulas to explain this figure. The Lorentz group is generated
by three rotation generators Ji and three boost generators
Ki.
The three rotation generators satisfy a closed set of commentators. Thus, the rotation group is a three-parameter subgroup of the Lorentz group. For a massive particle at rest, this rotation subgroup means the spin of the particle.
In his 1939 paper, Wigner constructed the following combinations of the generators.
They satisfy the closed set of commutation relations:
[ N1 , N2 ] = 0 ,
[ J3 , N1 ] = i N2 ,
[ J3 , N2 ] = - i N1 .Wigner then noticed that this set of commutation relations is identical to that for the E(2) group or the two-dimensional Euclidian group with with one rotational and two-translational degrees of freedom, while J3 corresponds to the rotation, and N1 and N2 to the translations along the x and y directions respectively.
While I was working with Wigner, we found out the cylindrical group also satisfies the same set of commutation relations.
For many years, it was not known that N1 and N2 generate gauge transformations applicable to the electromagnetic four-vector.
-
Genealogy of photons. Maxwell, Minowski, Einstein, and Wigner. You now know
where Wigner stands. Photos are from the public domain.
Also unknown to most of the physicists is the fact that the set of commutators given above is a Lorentz-boosted set of commutators for the rotation matrix.
- OK. We are done with the four-vector. How about the gauge-independent
Maxwell tensor? Yes, it is possible to construct them starting from the
SL(2,c) or two-by-two representation of the Lorentz group. You may look at
this book for this problem. I hope to be able to translate the calculations
given there into the language of webpages.
- This webpage was constructed by Y. S. Kim (2019.11.18).
- copyright@2019 by Y. S. Kim, unless otherwise specified.
- Click here for his home page.
- Einstein page, and
his Bern page.
- Wigner page,
and Princeton page.
- Travel page.
- Click here for his home page.
|