Evolution of the Hydrogen Atom
The hydrogen atom remained the same and will remain the same, not like the human race. As for the way we look it, there have been some evolutions.The hydrogen atom played its unique role as a physical model for bound states in quantum mechanics. Niels Bohr thought both the proton and electrons were point particles, and the proton was sitting at the center of the absolute frame.
-
In quantum mechanics, the picture of standing waves replaces the electron orbit,
creating discrete energy levels. Since we cannot accelerate the hydrogen
atom, there does not seem to be any urgent need for studying how the
orbit would appear to observers in different Lorentz frames.
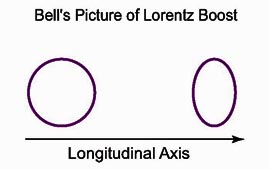
- In his book entitled "Speakable and Unspeakable in Quantum Mechanics"
(page71), J. S. Bell talks about how the electron orbit would appear if the
hydrogen atom moves and came up with this figure
this drawing. Yes, this Lorentz-deformed orbit is speakable,
but it is not observable, because the hydrogen atom cannot be accelerated.
The story is quite different if the electron is removed from the hydrogen atom. Indeed, the proton speed can reach the speed of light times 0.99999-- ( I do not know how many nines). Then what aspect of the hydrogen orbit can we study from those accelerated protons? What physics does the proton share with the hydrogen atom?
- The proton is one of the hadrons which are quantum bound states of quarks.
The hydrogen atom is a bound state.
- Like the hydrogen atom, hadrons have ground and excited states. Their
mass spectra are called Regge trajectories. Many people think God created
those Regge poles on the trajectories, but
Feynman pointed out
they represent degeneracies of the three-dimensional harmonic oscillators.
This aspect is not well known in spite of Feynman's presage.
- The question is whether we can entertain Bell's picture of Lorentz-deformed wave function using harmonic oscillator wave functions. Three-dimensional oscillator wave functions are separable in the Cartesian coordinate system, and thus the longitudinal coordinate can be treated separately.
- The proton is one of the hadrons which are quantum bound states of quarks.
The hydrogen atom is a bound state.
- In 1969, Feynman came up with the idea of partons. If the proton moves
with a velocity close to that of light, it appears as collection of free
partons behaving incoherently with external signals. Partons are routinely
assumed to be quarks in the papers on high-energy physics. The question is
whether these two different physical models are two different manifestations
of one covariant entity. This question has been addressed in this
webpage.

Form factor as a function of the (momentum transfer)2 . 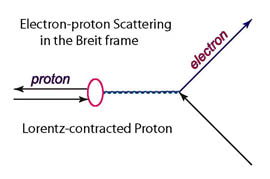
- Our next question is how the hadron would look if it gradually picks up.
In order to study the problem, let us consider the scattering of election
and proton. If the electron comes close to the proton, it emits a photon,
which is absorbed by the proton. If the proton is a point particle, we can
calculate the Rutherford formula for the scattering amplitude using one-photon
exchange Feynman diagrams.
But the Hofstadter experiment of 1955 showed the deviation from this formula indicating that the proton has a non-point charge distribution. I first heard about this in 1958 while I was doing a homework for my QM course at Carnegie Tech. I was a graduating senior then. Here is the homework problem.
- During this collision process, the momentum is also transferred. As the
momentum transfer increases, the deviation from the Rutherford scattering
becomes more prominent. This is consistent with an idea that the charge
of the proton is distributed, causing the deviation from the Rutherford
formula.
Thus, instead of a point in the Feynman diagram, we put a distribution function. Indeed, this is consistent with the quark model, where the proton is a bound state of the quarks. The Fourier transform of this density distribution with respect to the momentum transfer is called the proton "form factor."
- If the proton wave function is Gaussian, its charge distribution
is also Gaussian. If we take the Fourier transform of this Gsussian
function, the form factor should decrease like Gaussian, but this
is not what is happening in the world. The decrease is much slower.
The form factor goes through a polynomial decrease. This decrease is
known as the polynomial decrease.
What is the cause of this slower decrease? In order to explain this, let us exploit J.S.Bell's picture of Lorentz deformation. If we go to the Breit frame where the incoming momentum and outgoing momentum are in the opposite directions, as shown below. Then there are papers in the literature leading to the Lorentz-contracted distribution function like Bell's ellipse.
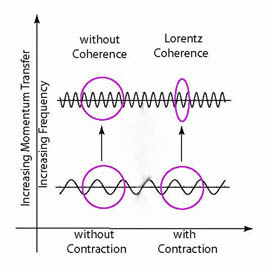
How does this figure explain the slower cutoff. The answer is the "coherence" as shown below. The width of the proton distribution decreases as the wavelength of the incoming photon decreases.
We can explain this also in terms of the overlaping wave functions in the momentum distribution function.
These figures are based on the published papers on this subject. They translate their writings into cartoons. See the power of cartoons in physics.
Click here for the latest article on this subject.
- Our next question is how the hadron would look if it gradually picks up.
In order to study the problem, let us consider the scattering of election
and proton. If the electron comes close to the proton, it emits a photon,
which is absorbed by the proton. If the proton is a point particle, we can
calculate the Rutherford formula for the scattering amplitude using one-photon
exchange Feynman diagrams.
- Click here for a hydrogen atom
moving with a speed close to that of light.
References
- H. Yukawa, Phys. Rev. {91}, 415 (1953),
- M. Markov, Suppl. Nuovo Cimento {3}, 760 (1956).
After the quark model.
- V. L. Ginzburg and V. I. Man'ko, Nucl. Phys. {74}, 577 (1965).
- A. L. licht and A. Pagnamenta, Phys. Rev. D {2}, 1150 (1970).
- K. Fujimura, T. Kobayashi, and M. Namiki, Prog. Theor. Phys. {43}, 73 (1970).
- R. P. Feynman, M. Kislinger, and F. Ravndal, Phys. Rev. D {3}, 2706 (1971).
- Y. S. Kim and M. E. Noz,
Symmerty [3], 16 (2011).
- Click here for a webpage dedicated to the history of this subject. This page will explain why some Japanese physicists say the string model was developed in Japan.

Yukawa with Feynman in Kyoto (1954). Courtesy of Saito Hayakawa. This idea has a long history. It is not uncommon to extract new ideas old papers.
Yet, the Lamb shift played the key role in the development of quantum electrodynamics, which is a Lorentz-covariant theory. This aspect is well known. what is not well known is the fact that the Lamb shift calculation still needs localized hydrogen wave functions which are constructed in Bohr's absolute frame. Do you know how the wave functions appear to moving observers?
|

|
|
Why is Feynman tilted?
Click here.
|
|

| |
|
- Click here for his
home page.
- Einstein page.
- Princeton page.
- His stories.