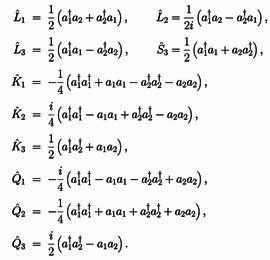Further Contents of Dirac's Squeezed States

Elvis changed the world by singing, on the wall of an American-style brasserie in Metz (France 2011). <

We can write a book on this subject. There are in fact many books on this subject. Many musicians attempted to write poems using musical tunes. They are called tone poems. Among those symphonic poets are Franz Liszt, Bedrich Smetana, and Jean Sibelius. The list could be endless.

However, we do not have to get that sophisticated to appreciate the power of poems in musical forms. When you sing your national anthem, you are reciting a poem both using its lyrics and its music. Yes, all songs are poems.

Elvis Presley was drafted to the U.S Army when I was an undergraduate student. He was so crazy that we all predicted that the Army would straighten him up. The net result was that Elvis changed the world. Perhaps he did not compose all those poems, but Elvis recited them well. In either case, poems are powerful, and they can change the world.

James Clerk Maxwell (1831-1878). This photo is in the public domain according the U.S. copyright law.

Click here to see where this portrait came from.

• Feynman and Wheeler communicated with each other by drawing cartoons. Indeed, we can express physical ideas very effectively in the form of cartoons. Feynman diagrams are cartoons. We often present our research results in the form of graphs and tables. Click here. You would agree that cartoons are graphic poems. Those graphic poems play important roles in physics.

• Is it possible to write poems using mathematical formulas? You all know how to write four Maxwell's equations. You will agree that this set of equations constitutes a poem, and you all know how this mathematical poem changed the world. If you did not know, click here for a story of how this wireless world was created.

• Paul A. M. Dirac has his own style of writing physics books and articles. You have all have read Dirac's book entitled The Principles of Quantum Mechanics. You will agree that this book is a book of poems.

  I bought the fourth edition of this book in 1958. In Secs. 55 and and 56 of this book, Dirac wrote a poem using permutations. Presumably, this poem was based on his earlier experience with the Fermi-Dirac statistics. By writing this poem, Dirac concluded that the permeation can be regarded as a dynamical variable. I have a webpage dedicated to this subject.

• Dirac used to say that formulas are more likely to produce profound physics. He was talking about himself. He produced new physics by writing mathematical poems. He wrote most of his mathematical poems using four-by-four matrices and harmonic oscillators.

  His Dirac equation for electrons and positrons was a history-making mathematical poem he wrote. Here he uses four gamma matrices, behaving like four vectors. From these matrices, we can construct axial vector, tensor, and pseudoscalar quantities. If we transform them into the Majorana representation, they all become 15 linearly independent traceless matrices with imaginary elements.

  Dirac also wrote many poems using harmonic oscillators in the step-up and step-down representation. In quantum mechanics, we start with the commutation relation between x and p variables. In the oscillator regime, we start with the operators

  [step-up = x + ip] and [step-down x - ip]

  Thus, Dirac's poems start with the uncertainty relation. I can write a long story about this, and let me stop here.

  If you are interested in Dirac's role in quantization of electromagnetic field, you may read a review article entitled

  On Dirac's New Method of Quantization,

  written by Wolfgang Pauli (1943). Since Pauli and Dirac were so important to each other, particularly in quantum statistics and quantization of fields, I would love to post Pauli's photo with Dirac, but I could not find one. Instead, I picked up Pauli's photo with another distinguished physicist named C. S. Wu. She is famous for performing the first parity violation experiment.

Dirac met people, but he seldom communicated with them. Why?

Two famous brothers-in-law.

• Dirac was Wigner's brother-in-law. Click here for an interesting story. However, did they communicate in physics. No, as far as I know. I used to talk to Wigner in his late years, and I talked to Dirac twice.

  In his 1949 paper, Dirac talks about a number of Wigner items contained in Wigne's 1939 paper on the Poincare group, but he does not make any reference to the Wigner's paper.

  One of the communication difficulties could have been that Dirac knew how to use four-by-four matrices but could not use anything smaller. On the other hand, in order to communicate with Wigner, you had to use two-by-two matrices. I know this. I was talking to Wigner.

  It was a rewarding experience for me to understand Dirac's four-by-four matrices in terms of the two-by-two matrices. Click here for one of the papers published along this line.

  Yukawa and Dirac (1953) (top), Dirac, Yukawa, and their wives (1955).

• Dirac met Hideki Yukawa in 1953, probably in New York. They met again in 1955. It appears that they met in Kyoto (Japan) in 1955. I assume they talked about physics. In 1953, Yukawa published a short paper entitled Structure and Mass Spectrum of Elementary Particles. II. Oscillator Model while he was visiting Columbia University. In 1955, Yukawa became more intensely interested in harmonic oscillators, while Dirac was using the oscillator language throughout his life.

  However, there does not seem to be any published material to indicate that they talked about harmonic oscillators. With one of my co-workers, I was able to able to compose a paper on what they could talked to each other on the oscillator issue, and published the paper in the Progress of Theoretical Physics, the Japanese Physics Journal established by Hideki Yukawa.

  In either case, both of them received their Nobel prizes for predicting new particles by writing mathematical poems.

  It is my pleasure to post a photo of Dirac and Yukawa (Kyoto 1955). Their wives are also in the photo. I never met Professor Yukawa, but I once received a letter from Mrs. Yukawa. Click here for her handwritten letter. In this letter, she talks about Mrs. Margit Dirac. I met Mrs. Dirac in 1978 (in Florida) and in 1988 (in Princeton). I met Paul A. M. Dirac in 1962 and in 1978.

• Dirac met Feynman in 1962. Three months later, in October, I met Dirac.

  In the fall of 1962, I was a first-year assistant professor at the University of Maryland. I was his driver when Dirac came spent two weeks in Maryland. I was fortunate enough to sit down with him to talk about physics for about 30 minutes.

  At that time, every physicist had to do follow order coming from Berkeley. I had to do "Regge poles" and "bootstraps." I did not like what they were doing, and I am the one who put an end to the bootstrapism in 1965. Click here for my story. This point was echoed by Geoffrey Chew who is known as the creator of bootstrap dynamics. Click here for Chew's paper.

  Jesus and Nicodemus, painting by Crijn Hendricksz (1616–1645) (top). Dirac and Feynman met in Poland (July 1962).

  Thus, when I met Dirac in 1962, I did not have any clear vision for myself. Indeed, it was Nicodemus meeting Jesus (Gospel of John). I asked Dirac what I should study. He said "American physicits" should spend more time to exploit contents of Lorentz covariance.

  If I translate this into a carton, Dirac was saying this. Many years later, I was fortunate enough to add something to this cartoon, and produce this cartoon. A similar cartoon is contained in my recent paper recent paper Marilyn Noz.

  However, the question was why Dirac was mentioning American physicists. This is an interesting question because Dirac seldom talked to American physicists.

  1. In Agust of 1963, the Physics Today carried this photo of Dirac and Feynman on its cover page. They mat at the International Conference on Relativistic Theories of Gravitation in July of 1962 (three months before I met Dirac in October), at the Jablonna Palace near Warsaw, Poland. I suspected that Dirac was talking about Feynman when he mentioned "American physicsits" to me. This photo clearly indicates there was a communication gap between these two great physicists.

  2. In 1971, Feynman published this paper with his students. It then became clear to me that Dirac indeed was talking about Feynman, when he told me American physicists should study Lorentz covariance.

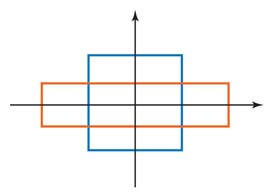
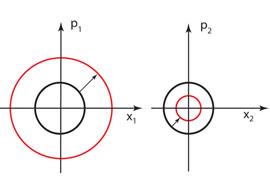
  Two-by-two matrices can do this kind of transformations. They can be called "squeeze" transformations.

• This gave me enough motivation to build a research program: to put Dirac and Feynman into one box. I talked about this issue many times before, and I do not have to explain this again. You can go back to my earlier page on this subject.

• In so doing, I found out why they could not talk to each other. Let us assume that they knew how to use two-by-two matrices for rotations. If they knew those matrices can also perform squeeze transformations, like this, or like this they could have communicated, and the physics world could have have been quite different. It is much easier to understand Dirac's papers and Feynman's papers in terms of those squeeze transformations.

• Let us finally talk about Nicodemus. I mention him often during my conference talks. I talk about him when I mention my encounter with Dirac in 1962. I then see blank faces from the audience.

  Nicodemus is mentioned in the Gospel of John, but he appears to be totally irrelevant to physics issues. Yet, I could be talking about you whenever I mention his name. In order to emphasize this point, let me tell you that I am not the first person who attempted to identify himself with Nicodemus.

• You all know Michelangelo was a creative person, and you would not mind becoming as creative as he was, even though he was in a different field. Among many of his sculptures, the most famous one is the Pieta (Mary holding the dead body of Jesus) in Vatican's Saint Peter's Basilica.

  There is another Pieta Michelangelo constructed in his later years. It is said that he constructed this Pieta to decorate his tomb in Rome, but it is now housed in in the Museo dell'Opera del Duomo in Florence.

  As you can see, Nicodemus is the most prominent figure in the sculpture. Here, Michelangelo portrayed himself as Nicodemus. Why did he do so? You find out the answer. The story could be talking about you.